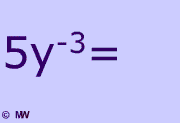Formula for Negative Exponents
The general formula for rewriting negative exponents as a positive exponent is :
$
\boxed{ x^{- \red a} = \frac {1}{x^{\red a}} }
$
Examples of rewriting negative examples as positive
$
\text{ 1) } 5^{- \red 2} = \frac {1}{5^{\red 2}}
\\
\text{ 2) } (3x)^{- \red 2} = \frac {1}{(3x)^{\red 2}}
$
Practice Problems Simplifying Negative Exponents
Use the negative exponent formula to rewrite each formula so that there all exponents are positive.
Problem 1
Problem 2
Problem 3
Related:
